关键词 > JACOBIFIELD
PROBLEM SET 4: JACOBI FIELD
发布时间:2024-06-15
Hello, dear friend, you can consult us at any time if you have any questions, add WeChat: daixieit
PROBLEM SET 4: JACOBI FIELD
DUE: JUNE 4, 2024
Instruction: Please work on at least Five problems of your interest, and in each problem you are supposed to work on at least TWO sub-problems.
1. [Jacobi fields for manifolds with constant sectional curvature along γ]
![]() Let (M, g) be a Riemannian manifold, and γ : [0, l] → M a normal geodesic, where we assume l ≠ kπ/√κ if κ > 0. Suppose M has constant sectional curvature κ along γ, i.e. i.e. K(Πγ(t)) = κ for any 2-dimensional plane Πγ(t) > γ˙(t).
Let (M, g) be a Riemannian manifold, and γ : [0, l] → M a normal geodesic, where we assume l ≠ kπ/√κ if κ > 0. Suppose M has constant sectional curvature κ along γ, i.e. i.e. K(Πγ(t)) = κ for any 2-dimensional plane Πγ(t) > γ˙(t).
(a) Prove: The Jacobi field V along γ with V (0) = 0 and V (l) = Xl ∈ (γ˙(l))丄 is
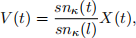
where X is the parallel vector field along γ with X(l) = Xl.
(b) Given any X0 ∈ Tγ(0)M and Xl ∈ Tγ(l)M, find the Jacobi field with V (0) = X0, V (l) = Xl.
2. [Characterizing constant curvature via Jacobi field]
We say a vector field Y along γ is almost ![]() arallel if there is a smooth function f so that Y (t) = f(t)X(t), where X is parallel along γ . As we have seen, any normal Jacobi field along a geodesic on a constant curvature space is almost parallel.
arallel if there is a smooth function f so that Y (t) = f(t)X(t), where X is parallel along γ . As we have seen, any normal Jacobi field along a geodesic on a constant curvature space is almost parallel.
(a) Suppose γ : [0, l] → M is a geodesic in (M, g) with γ(0) = p, so that any normal Jacobi field along γ is almost parallel. Let V C TpM, and V0 a small neighborhood of 0 in V such that expp : V0 → N = expp(V0) is a diffeomorphism. Suppose γ([0, l]) C N. Prove:
P0(γ),l (V) = Tγ(l)N.
(b) Suppose for any geodesic γ with γ(0) = p, any normal Jacobi field along γ is almost parallel. Prove: for any pairwise orthogonal vectors u,v, w ∈ TpM , (R(u,v)u,w〉= 0.
(c) Suppose m ≥ 3. Prove: If any normal Jacobi field along any geodesic in M is almost parallel, then M has constant sectional curvature.
3. [Square of distance in normal coordinates]
Consider two geodesics γ1 (t) = expp(tv) and γ2 (t) = expp(tw) emanating from p. Let g(s) = d2 (γ1 (s),γ2 (s)). To estimate L(s) for s small, consider the variation
f(t, s) = σs(t) := expγ1(s) (t exp−1γ1(s) (γ2(s))).
Let ft(t,s) = dft,s(∂/∂t) and fs(t,s) = dft,s(∂/∂s) as usual. Note that for s small, σs is the minimizing geodesic from γ1 (s) to γ2 (s), and g(s) = Ⅱσ˙ s(t)Ⅱ2 = Ⅱft(t,s)Ⅱ2 . [In what follows,
although we use usual notation for the connection, they should be understood as the induced connection.]
(a) Show that ft(t,0) = 0, fs(0, s) = γ˙ 1(s), fs(1, s) = γ˙ 2(s).
(b) Show that (▽∂/∂tft)(t,s) = 0, (▽∂/∂sfs)(0,s) = 0, (▽∂/∂sfs)(1,s) = 0.
(c) Show that for each fixed s, fs(t,s) is a Jacobi field along γs, and (▽∂/∂t▽∂/∂tfs)(t,0) = 0. Conclude that fs(t, 0) is linear and thus fs(t,0) = v + t(w — v).
(d) Show that g′ (0) = 0, g′′ (0) = 2|v — w| 2 .
(e) Show that (▽∂/∂t▽∂/∂t▽∂/∂sfs)(t,0) = 0,which implies (▽∂/∂sfs)(t,0) is linear in t. Conclude that (▽∂/∂sfs)(t,0) = 0, (▽∂/∂t▽∂/∂sfs)(t,0) = 0, (▽∂/∂s▽∂/∂sft)(t,0) = 0, and g′′′ (0) = 0.
(f) Show that g′′′′ (0) = 8Rm(v,w,v, w).
(g) Conclude that g(s) = |v − w| 2 s 2 + 3/1Rm(v, w, v, w)s 4 + O(s 5 ) and thus
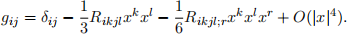
4. [Expansion of metric in normal coordinates: next term]
(a) Prove: In a Riemannian normal coordinates, near p we have
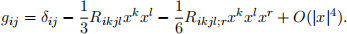
What can you say about the coefficients of higher order terms?
(b) Expand det(gij) up to order 3 near p.
(c) Prove Bianchi Identity II using normal coordinates
(d) Prove: A chart is a Riemannian normal coordinate system if and only if for any i, gijxj = xi.
5. [Locally symmetric space]
Let (M, g) be a locally symmetric space, i.e. if ▽X R = 0 for all X ∈ Γ∞(TM). Let γ : [0, a] → M be a geodesic in M with p = γ(0), Xp = γ˙(0).
(a) Let X,Y,Z be vector fields that are parallel along γ . Prove: R(X, Y)Z is also parallel along γ .
(b) Define a linear transformation KXp : TpM → TpM by
KXp(Yp) = R(Xp,Yp)Xp.
Prove: KXp is self-adjoint.
(c) Let λ 1 , ··· ,λm be eigenvalues of KXp, with corresponding eigenvectors e1 , ··· , em ∈ TpM.
Let ej(t) be the parallel transport of ej along γ . Prove: for all t ∈ [0, ∞ ), we have
Kγ˙(t)(ei(t)) = λiei(t), i = 1, ··· ,m.
(d) Let X(t) = Xi(t)ei(t) be a Jacobi field along γ . Show that the Jacobi equation becomes
X(¨)i(t) + λiXi = 0, i = 1, ··· ,m.
(e) Conclude that the conjugate points of p along γ are given by
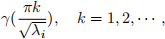
where λi’s are positive eigenvalues of KXp .
6. [More on cut locus] Let (M, g) be complete.
(a) Prove: The function f : SM → R U {∞} defined by
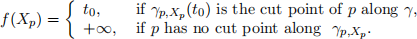
is continuous.
(b) For any p ∈ M, Cut(p) is closed.
(c) M is compact if and only if Cut(p) is nonempty and compact for any p ∈ M.
(d) Let Σ(p) = {tXp | Xp ∈ SpM, 0 ≤ t < f(Xp)} . Prove: expp : Σ(p) → expp(Σ(p)) is a diffeomorphism.
(e) Prove: M = expp(Σ(p)) ∪ Cut(p) and expp(Σ(p)) ∩ Cut(p) = ∅ .
(f) We call a point q ∈ M a regular cut point if there exists at least two minimal geodesic from p to q. Prove: The set of regular cut points is a dense subset of Cut(p).
7. [Smoothness of distance function]
For any p ∈ M, consider the distance square function
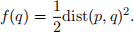
(a) For (M, g) = (Sm , gSm) the standard sphere, is f a smooth function? (b) Argue that f is smooth on M\Cut(p).
(c) For any q ∈ M\Cut(p), find (∇f)(q).
(d) For any q ∈ M \Cut(p) and Yq ∈ TqM, let X be a Jacobi field along γq , the mini- mal geodesic from p to q, so that X(0) = 0, X(dist(p,q)) = Yq . Prove: ∇2f(Yq,Yq) = dist(p,q)⟨∇γ˙q (dist(p,q))X, X(dist(p,q))⟩ .
(e) Prove: f is not C1 function at regular cut points.
(f) Can f be everywhere smooth on M if M is compact?
8. [Convex Functions on Riemannian Manifolds]
• Let (M, g) be a Riemannian manifold. A function f : M → R is said to be a convex function if for any geodesic γ : [a,b] → M, the function f ◦ γ : [a,b] → R is convex.
(a) Prove: If f is a convex function on M, then for any c ∈ R, the sublevel set Mc = {p ∈ M | f(p) < c} is a totally convex subset of M.
(b) Prove: Iff is smooth,thenf is convex if and only if its Hessian ∇2f is positive semidefinite.
(c) Let p ∈ M be an arbitrary point, and dp(q) = dist(p,q) is the distance function from p.
Prove: There exists an neighborhood U of p so that the distance square function dp(2) is convex on (U, g).
• Now suppose (M, g) is a complete simply-connected Riemannian manifold with non-positive sectional curvature.
(d) Prove: the distance square function
d2 : M × M → R, d2 (p,q) = [dist(p,q)]2
is convex on M × M.
(e) Conclude that for any p ∈ M, the function dp(2) is a convex function on M.

