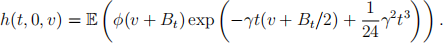关键词 > MA482
MA 482 STOCHASTIC ANALYSIS FOURTH YEAR EXAMINATION: SUMMER 2021
发布时间:2024-05-20
Hello, dear friend, you can consult us at any time if you have any questions, add WeChat: daixieit
MA 482
FOURTH YEAR EXAMINATION: SUMMER 2021
STOCHASTIC ANALYSIS
Time Allowed: 3 hours
Read all instructions carefully. Please note also the guidance you have received in advance on the departmental ‘Warwick Mathematics Exams 2021’ webpage.
Calculators, wikipedia and interactive internet resources are not needed and are not permitted in this examination. You are not allowed to confer with other people. You may use module materials and resources from the module webpage.
ANSWER COMPULSORY QUESTION 1 AND TWO FURTHER QUESTIONS out of the three optional questions 2, 3 and 4.
On completion of the assessment, you must upload your answer to Moodle as a single PDF document if possible, although multiple iles (2 or 3) are permitted. You have an additional 45 minutes to make the upload, and instructions are available on the departmental ‘Warwick Mathematics Exams 2021’ webpage.
You must not upload answers to more than 3 questions, including Question 1. If you do, you will only be given credit for your Question 1 and the irst two other answers.
The numbers in the margin indicate approximately how many marks are available for each part of a question. The compulsory question is worth 40 marks, while each optional question is worth 30 marks.
Throughout this exam unless stated otherwise you may assume,
(Ω ; F; r) is a probability space,
(Ft )t 0 is a iltration satisfying the usual conditions,
(Bt )t 0 is a (Ft )t 0 Brownian motion.
COMPULSORY QUESTION
1. This question is about the Ornstein-Uhlenbeck process. Throughout this question X is the solution to the SDE
dXt = -λXtdt + σdBt, (1)
where λ > 0, σ > 0.
a) Find d(eγtXt ) for γ 2 R.
b) For which values of γ is eγtXt a martingale?
c) By choosing γ show that
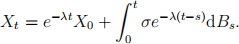
d) When X0 = x compute E(Xt ).
e) Let Yt = eλtXt. What is the quadratic variation of Yt?
f) Using the Dubins-Schwarz theorem, write Yt in the form
Y0 + WF (t) ,
where W is a Brownian motion and F is a function of time.
g) Use part (f) to write Xt in the form
H(t, X0 ) + G(t)WF (t).
h) Write down the Kolmogorov forward equation for the law of the solution to the SDE (1).
i) Using the Kolmogorov forward equation ind the law of a steady state solution to the SDE. How does this relate to your answer to part (g)?
OPTIONAL QUESTIONS
2. The aim of this question is to solve the SDE
dYt = α(1 - Yt )dt + βYtdBt.
a) First, ind an Ito process Zt such that
d(YtZt ) = αZt.
b) Write down the equation that will be satisied by the law of the steady state of this equation. Solve the steady state equation separately for y > 0 and y < 0.
c) Looking at the explicit solution of the SDE, state whether Yt is going to tend
to be mostly negative, mostly positive or neither as t ! 1. Use this to ind a condition on α and β for the steady state to exist and write down the steady state without explicitly calculating the normalising factor. Explain how the condition relates to the behaviour of Zt.
3. For a time t > 0 and an integer n let tj = (j2-n ) ^ t, j = 0, . . . , L2nt」.
a) Suppose you want to approximate the Ito process Xt = X0 +10(t) Usds+10(t) σsdBs ,
where U, σ are continuous stochastic processes and σ is an admissible integrand and an Ito process. Which of the following approximations should you use?
(i)
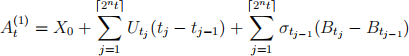
(ii)
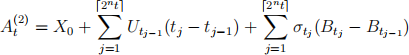
Justify your answer.
b) For both approximations, identify the limit (without proving the convergence) as n ! 1 of the approximation in L2 (Ω) in the following cases:
(i) When Us = 0 and σs = Bs, ∀s ≤ t.
(ii) When Us = -1 and σs = BsAs ≤ t.
(iii) For general U, σ leaving your answer in terms of time integrals, Ito integrals and quadratic covariations.
(iv) When Us = 0 and σs = Bs(2) - s,As ≤ t. Find any quadratic covariation terms explicitly.
4. a) Explain why10(t) Bsds is a Gaussian random variable.
b) Show that coU (Bt , 10(t) Bsds) = t2 /2 and that E ( (10(t) Bsds)2 ) = t3 /3. Hence ind the joint density fBt ,'0(t) Bsds(g, z) of (Bt , 10(t) Bsds). Leave your answer in terms of matrix determinants and inverses without explicitly computing them.
c) For γ > 0, and φ E C0(2)(R) (twice continuously diferentiable and vanishing at ininity), consider the PDE
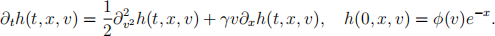
Where x E R, U E R, and t > 0. Write the solution h(t, x, U) in the form Ex,v (Zt ) where Zt is a stochastic process you should specify. Give the name of the theorem you are using.
d) Using your answer to parts (b) and (c) write h(t, x, U) in the form
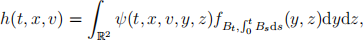
where you should ind ψ explicitly.
e) Using the fact that the conditional density of 10(t) Bsds given Bt is
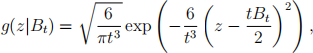
show that